Introducing kinematics 1.f constant velocity answers, a comprehensive guide to understanding the motion of objects with unwavering speed. This article delves into the intricacies of constant velocity, providing insightful explanations, practical examples, and essential equations to empower your understanding.
Embark on a journey through the world of kinematics, where we unravel the significance of constant velocity in physics, explore its applications in various fields, and equip you with the tools to solve complex motion problems.
Kinematics
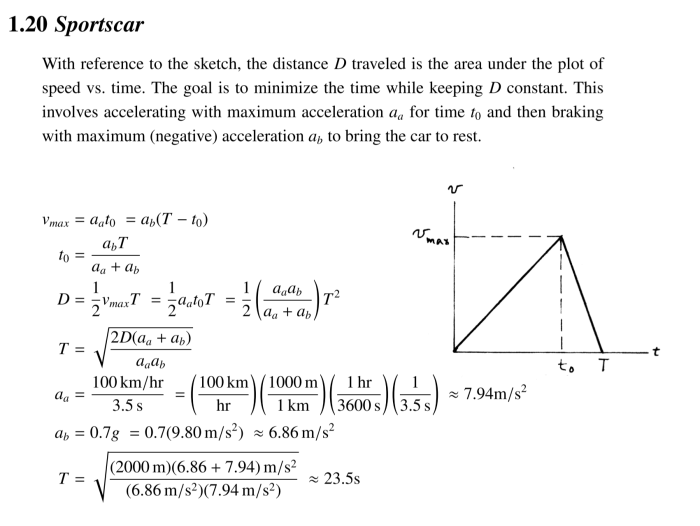
Kinematics is the branch of physics that describes the motion of objects without considering the forces that cause the motion. It deals with the relationships between displacement, velocity, acceleration, and time. Kinematics is essential in understanding the motion of objects in various fields, such as engineering, sports, and transportation.
Kinematics differs from kinetics, which deals with the forces that cause motion. Kinematics provides the mathematical framework for describing motion, while kinetics explains why objects move the way they do.
Applications of Kinematics, Kinematics 1.f constant velocity answers
- Predicting the trajectory of projectiles, such as rockets and bullets
- Calculating the velocity and acceleration of objects in motion, such as cars and airplanes
- Designing amusement park rides to ensure safety and excitement
- Analyzing the motion of celestial bodies, such as planets and stars
Constant Velocity
Constant velocity is a type of motion in which the velocity of an object does not change over time. This means that the object is moving at a constant speed in a straight line.
Constant velocity is calculated using the following formula:
v = d/t
where:
- v is the velocity in meters per second (m/s)
- d is the displacement in meters (m)
- t is the time in seconds (s)
Examples of objects moving with constant velocity include:
- A car traveling at a constant speed on a straight road
- A ball rolling down a hill at a constant speed
- A satellite orbiting the Earth at a constant speed
Equations of Motion for Constant Velocity
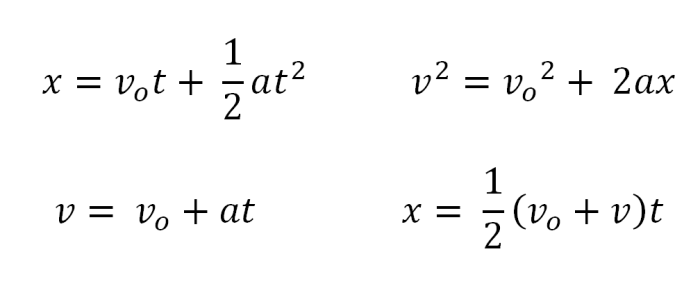
The three equations of motion for constant velocity are:
- v = d/t
- d = v*t
- t = d/v
The first equation calculates the velocity of an object, the second equation calculates the displacement of an object, and the third equation calculates the time taken for an object to travel a certain distance.
These equations can be used to solve a variety of kinematics problems. For example, if you know the displacement and time of an object, you can use the first equation to calculate the velocity of the object.
Applications of Constant Velocity Equations
Constant velocity equations are used in a variety of fields, including:
- Engineering: To design and analyze machines and structures
- Sports: To analyze the motion of athletes
- Transportation: To design and operate vehicles
For example, constant velocity equations can be used to calculate the speed of a car, the distance traveled by a train, or the time it takes for a plane to reach its destination.
| Field | Application | Equation |
|---|---|---|
| Engineering | Calculating the speed of a conveyor belt | v = d/t |
| Sports | Calculating the distance run by a sprinter | d = v*t |
| Transportation | Calculating the time it takes for a train to travel between two stations | t = d/v |
Answers to Common Questions: Kinematics 1.f Constant Velocity Answers
What is the definition of constant velocity?
Constant velocity refers to the motion of an object that maintains a consistent speed and direction.
How is constant velocity calculated?
Constant velocity is calculated by dividing the displacement (change in position) of an object by the time taken for that displacement.
What are the three equations of motion for constant velocity?
The three equations of motion for constant velocity are: